Oscillating system shock spectrum and Q – factor
Shock spectrum is a diagram of resonant bodies with a single degree of variance maximum response to external impact, arranged by resonant bodies frequencies.
This is a short and precise definition, however it arises a lot of questions due to the fact that it is difficult for people to imagine even one resonant body with a single degree of variance while we are considering resonant bodies system.
If we try to explain this notion at the elementary level, we should be precise and consistent so that to give you an idea of the notion in question. It is much easier in the case of tangible objects – it is good enough simply to demonstrate them. For abstract notions it is impossible – but we shall try.
Resonant body with a single degree of variance
Let us imagine an object oscillating only in a single axis – this is the resonance body with a single degree of variance. Springs and pendulums are resonant bodies with a single degree of variance. In spite of the fact that the nature of their oscillations is different, similar formulas are used to describe them. They have their own frequency and a resonance frequency. Even though these are two different frequencies, they are combined into a single one for the purpose of convenience. Resonance frequency is the frequency of an external force impact resulting into maximum oscillations amplitude. Fundamental frequency is the frequency of dying-out vibrations produced when the external impact is over and the system is loosing energy while restoring equilibrium position.

Let us imagine a pendulum system that we know since the childhood – a swing set.
Pendulum resonance frequency does not relate to the load weight (whether the load is represented by a little girl or her dad) – in depends only on a suspension length. The longer is the suspension, the higher will be the resonance frequency. Hence, the higher the swing set is, the longer will be the swing cycle.
At first the child applies a lot of effort with random frequency trying to start swinging. Then he realizes the necessary pace to start swinging and understands that the efforts should be applied at the peak positions of the swing cycle.
From scientific point of view the swing amplitude increases when the outer force impact coincides with resonance frequency thus causing the system to enter the resonance.

The child is not strong enough to swing too high. At a certain moment all his efforts are used to maintain the swing on one and the same level instead of increasing it. In this case all the energy will be spent for overcoming suspension friction and air resistance. If we suppose that the amount of efforts applied for each swing cycle is the same (work function A), then, after having achieved maximum swing rate for n cycles, the amount of energy wasted will be:
W=n×A,
this energy will transform into the swing set energy (some part of it will be dissipated, but it is non-essential).
After that all the energy will be dissipated during one swing cycle:
A=E×d
After having discussed this moment we can move forward to the Q-factor notion
Q – factor
Q – factor – is an oscillating system parameter, that determines the resonance width and shows how far the system energy amount exceeds the energy loss for a single oscillation period. General Q-factor calculation for any oscillating system is calculated by the following formula:

f0 – resonance frequency;
W – system energy;
Pd – dissipated power;
Ed – the amount of power dissipated for a single oscillation period;
A – work function performed by external force for a single oscillation period;
n – number of oscillations performed by the system prior to achieving the maximum oscillation amplitude.
This formula allows us to arrive at an important conclusion:
The higher is the oscillating system Q-factor, the more system oscillations will be caused by external force inpact before there will be achieved the maximum amplitude.
I.e. the higher the swing set is (pendulum length is proportional to the Q-factor), the more oscillations are required to swing it. External force amount determines only the oscillations amplitude produced by the system. If it is the dad who swings the system (with more power, and, hence, energy), th swing set will rise higher, but the maximum amplitude will be achieved after almost the same number of oscillations. However, the dad has to be cautios, otherwise the child may feel sick or the swing set can break.
Basic frequency
As kids grow, swinging up and down becomes boring for them – so they jump off the swing set trying to get higher (the good thing about it is that the playgrounds are normally covered with sand). After the jump the swing set does not experience impact of the external force and the dad stops to swing empty swing set. Gradually the swing set amplitude decreases and the swing set stops. The interval between two deviations of the swing set (or a pendulum, resonating body, signal, etc) is called oscillations period and the reverse value – oscillations frequency.
Some oscillating systems stop soon – just after a couple of oscillations (most of the swing sets make up to 7 oscillations), while other oscillating system have long oscillation period (bells are oscillating systems as well). The speed of oscillations damping is a very important factor called damping decrement.
Damping decrement
Damping decrement or a logaritmic decremet is a non-dimensional physical value describing oscillating process amplitude decrease which is equal to the base ratio logarithm of two consequent (or separated by several periods) oscillation value amplitudes:

Damping decrement is equal to the decay constant:

Damping decrement can be used for calculating of one more value – damping constant:


Damping ratio
Damping ratio is a rated value which can be calculated by means of damping oscillation diagram. For oscillating systems having viscous friction (resistive force is proportional to the speed) the key physical value wihich determines oscillation characteristics is the damping ratio.
 , where
, where
c – resistive force ratio ;
k – elasticity ratio;
m – movable weight ratio.
If damping ratio is <1, the oscillating system will be dampening softly. The higher is the ratio, the longer the oscillations will last. If damping ratio is ≥1, there will be no oscillations, the system will be tending to a stable position. Thus, the door brakes damping ratio is ≥1, which allows the door to be closed without slamming the door aperture. The door brakes in a subway are set for damping ratio <1.
Damping ratio and Q-factor are interrelated by the following formula:

From the formula above it follows that the oscillating system Q-factor is proportional to the damping ratio. The lower the damping ratio is, the less energy is spent with each oscillation and the more oscillations will be performed by the system before it stops. This very conclusion will be useful for our further discussion.
For swing sets, pendulums and other systems having low basic frequency (and, hence, large oscillation period) it is easy to calculated the amount if oscillations. However, if we consider bells, beams and other systems having high basic frequency, it is impossible to make a fast estimation of the remaining oscillations amount.
Resonant bodies system
If we imagine several resonant bodies with different basic frequency values, but the same damping ratio, they will form that very resonant system which has been mentioned in the beginning of the article. Let us imagine a playground with a swing sets of different dimensions and similar construction. The size of a swing set will determine their basic frequency while their structures and materials determine the damping ratio. Thus, they will have different different basic frequencies and identical damping ratio.

Let us suppose that all the swing sets are simultaneously exposed to excitation force impact causing them to swing. In this case the maximum oscillations amplitude that can be achieved by the swings sets will be the maxium response value. Similar impact can be caused by an earthquake. If we arrange maximum response values by basic frequencies increase, then the diagram obtained will represent the shock spectrum. In the case of an earthquake the shock spectrum is termed as a response spectrum.
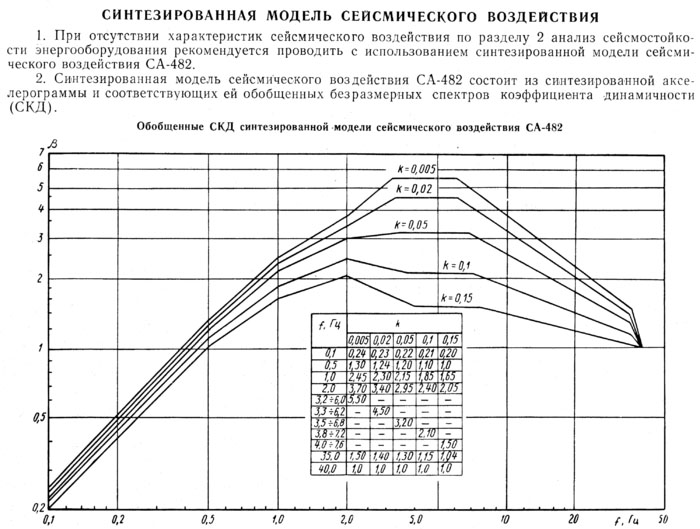
As we have already mentioned, maximum response of some resonant bodies is not necessarily to be achieved at maximum force value point – it may occur at any other moment. This moment depends on harmonic values present in the signal and their duration. Even in the case if the system is exposed to harmonic signal with even frequency, all the resonant bodies will be affected by it. Maximum response amplitude will be produced by resonant body having basic frequency value close to that of oscillation frequency. This is particularly evident from the resonance diagram.
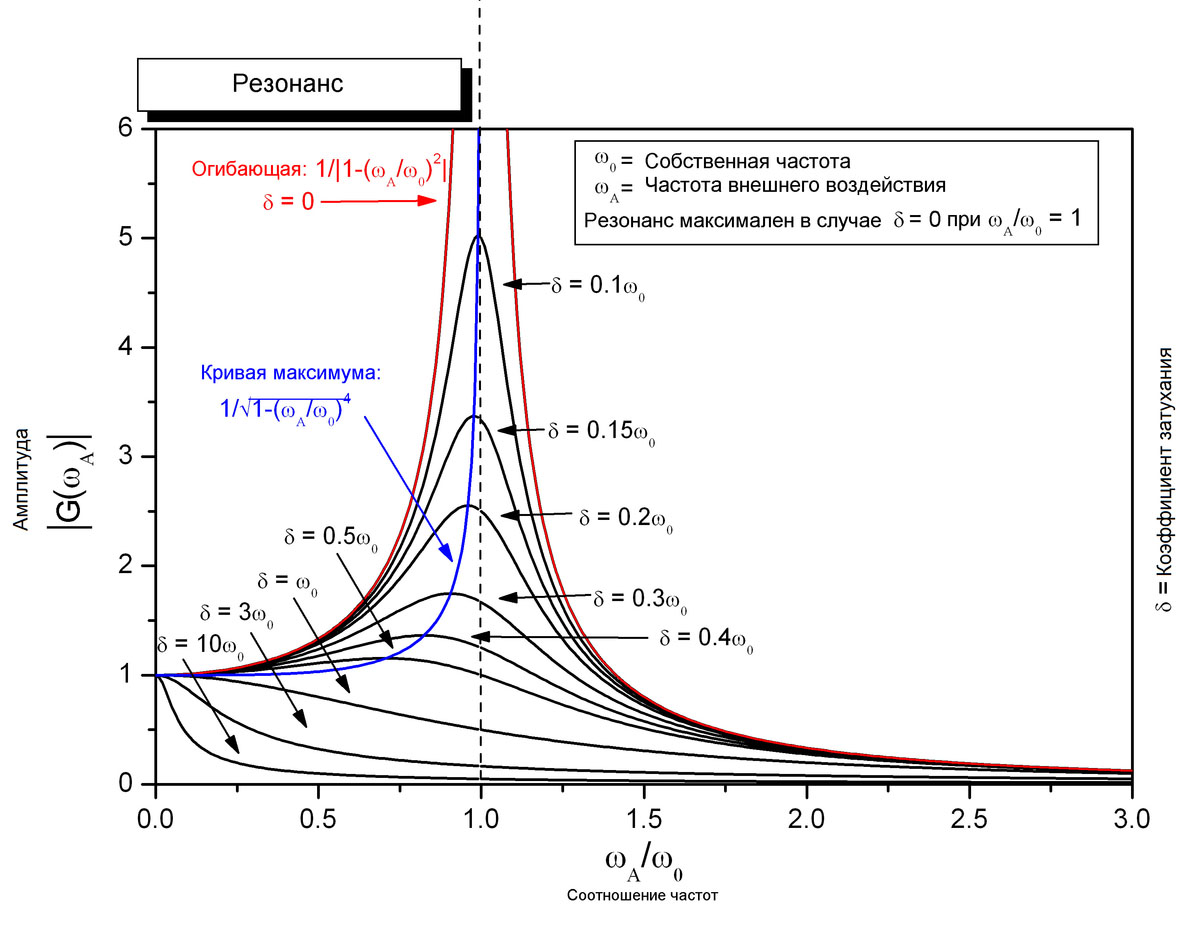
If we consider short impact (instead of stable oscillations), the situation will be quite different. Let us produce an impact to the resonant bodies system with a pulse consisting of several sine signals periods with 1000 Hz frequency from half-sine up to 10 periods. Damping ratio of all resonant bodies is 0.05, Q-factor – 10.
As we can see from the shock spectrum diagram, longer impact time leads to an increase of resonance system maximum response; besides, the frequency causing the maximum response is close to that of the resonant body. Now the question is: “Why do pulses with small periods number cause a stronger response in resonant bodies with frequency values exceeding that of the impact pulse?”. In order to answer this question we should have a closer look at the resonance diagram above.
The diagram shows dependence of resonant body response to constant amplitude impact with a variable frequency. The diagram clearly shows that the resonant bodies with a low Q-factor enter resonance at a frequency lower than that of the resonant body. As the resonant body Q-factor increases, the resonance peak becomes higher while its frequency tends to the resonant body basic frequency.
For the shock spectrum the situation is quiet different. Input signal frequency remains unchanged while resonant bodies frequencies are changing. Each resonant body Q-factor has a limit, however, impact duration allows to affect all the resonant bodies, hence, Q-factor of each resonant body will be determined by signal periods amount (≤10).
In the case if the resonant body frequency exceeds that of the input signal, then wa/w0 < 1 - thus, the response does not go below the input signal amplitude. That means that the pendulums with short suspension do not swing due to low-frequency impact and simply follow it. Hence, compact buildings (like call-boxes, boothes, etc) do not have internal stress in the case of an earthquake and simply follow its wave. If the resonant body frequency is lower, then the ratio is wa/w0 > 1 and the response amplitude value is decreasing together with resonant body frequency decline. That means that the pendulums with long suspension do not swing beacuse of high frequency impacts. Due to this reason high buildings (consisting of large sections) do not react to paving brakers impact irrespective of their amount.
Let us analyze resonance diagram corresponding to δ=0.5w0 – it will correspond to shock-response spectrum for half-sine period (the only difference is that the diagram will have “reflected” representation). Signals with a bigger number of periods will be described by diagrams with higher Q-factor. If we align several shock spectrum diagrams in the same axes, there will be produced a diagram similar to the resonance diagram shown above, but “reflected” by 180 degrees.
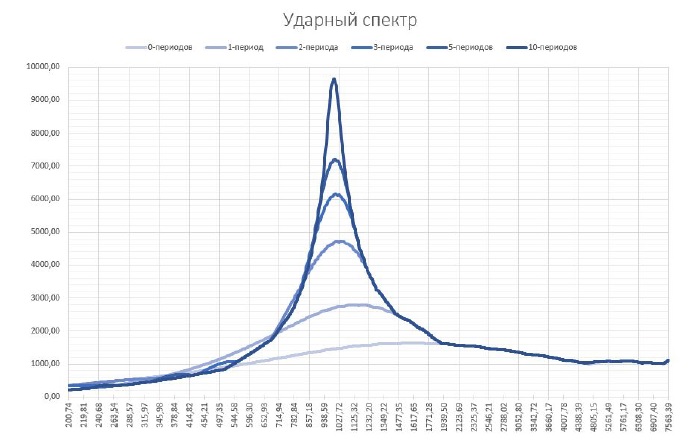
Conclusions
As a conclusion we should say that the shock spectrum is a good representation of object’s inner state and condition. Thus, for small objects it is possible to estimate “inner resonant objects system” condition by analyzing their shock spectrum. Materials fatigue, inner cracks and other defects correspond to changes in this “inner resonant objects system”. Normally such processes result in the change of basic and resonant frequencies and sometimes lead to oscillating circuits Q-factor decrease.
Thus, the church bells eventually “lower” their sound and pitch due to decrase in basic frequency (also referred to as an undertone). Brass “aging” is a similar process [1]. In the case if there appears a crack in the bell (for example, because of a frost), it will lead to a decrease of its Q-factor.


Tested object can be described as a resonant bodies system or as a set of strings (similar to those inside of a piano) having certain sound. As the tested object has been in use for a while, the sound change allows to detect inner changes. Thus, in the applicable technical requirements it is recommended to measure and compare the FR characteristics of the tested object prior to and after the test perfomance. Another example is the base frequency change of a building – in the case if the base frequency value changes more than twice upward or downward, the authorities conclude that the building is in a critical condition.
In the case of an earthquake it is the earthquake shock spectrum that is measured – it allows the scientists to estimate buildings and structure damage [2]. A range of most destructive frequencies is calculated for each type of the constructions – the more earthquake frequencies coincide with the specified range, the more damage will be caused to a particular building.
When designing building structures in earthquake-prone areas special oscillation damping elements are introduced into buildings design. Damping systems are intended for reducing impact of the most dangerous frequencies.These frequencies are determined by means of seismic record analysis throughout the record period. Upon design works completion the buildings are exposed to a model eartquake test impact [3].

References
- https://www.muszone.ru/articles/23.html
- https://ru.wikipedia.org/wiki/%D0%9C%D0%BE%D0%B4%D0%B5%D0%BB%D1%8C_%D1%81%D0%B5%D0%B9%D1%81%D0%BC%D0%B8%D1%87%D0%B5%D1%81%D0%BA%D0%BE%D0%B3%D0%BE_%D0%B2%D0%BE%D0%B7%D0%B4%D0%B5%D0%B9%D1%81%D1%82%D0%B2%D0%B8%D1%8F_%C2%AB%D0%A1%D0%90-482%C2%BB
- https://ru.wikipedia.org/wiki/Сейсмостойкое_строительство
- https://www.iris.edu/hq/inclass/video/building_resonance__boss_model_construction__use
P.S.
Finally we can move from mechanical and seismical sphere to social aspects. In society there exist similar processes called “public response”. When some event has a strong hold on the minds (in other words, “strikes a deep chord in the hearts of people”), it calls a public response. Such events may cause people to act (for example, to write a letter to a TV-channel or a newspaper editorial office). People who receive such letters can sort them out and analyze public response.
This social event may not be identical to the resonance and shock spectrum in mechanics, but it, however, allows to give a simplified notion of mechanical resonance to many people.
